Rings in which Every Element is a Sum of Two Tripotents

Abstract

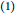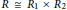Let $R$ be a ring. The following results are proved. $\left( 1 \right)$ Every element of $R$ is a sum of an idempotent and a tripotent that commute if and only if $R$ has the identity ${{x}^{6}}\,=\,{{x}^{4}}$ if and only if $R\,\cong \,{{R}_{1}}\,\times \,{{R}_{2}}$, where 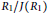${{{R}_{1}}}/{J\left( {{R}_{1}} \right)}\;$ is Boolean with 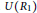$U\left( {{R}_{1}} \right)$ a group of exponent $2$ and ${{R}_{2}}$ is zero or a subdirect product of 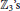${{\mathbb{Z}}_{3}}^{,}s$. 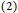$\left( 2 \right)$ Every element of $R$ is either a sum or a difference of two commuting idempotents if and only if 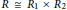$R\,\cong \,{{R}_{1}}\,\times \,{{R}_{2}}$, where 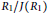${{{R}_{1}}}/{J\left( {{R}_{1}} \right)}\;$ is Boolean with 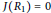$J\left( R \right)\,=\,0$ or 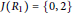$J\left( R \right)\,=\,\left\{ 0,\,2 \right\}$ and ${{R}_{2}}$ is zero or a subdirect product of 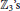${{\mathbb{Z}}_{3}}^{,}s$. 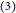$\left( 3 \right)$ Every element of $R$ is a sum of two commuting tripotents if and only if 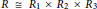$R\,\cong \,{{R}_{1}}\,\times \,{{R}_{2}}\,\times \,{{R}_{3}}$, where 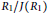${{{R}_{1}}}/{J\left( {{R}_{1}} \right)}\;$ is Boolean with 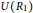$U\left( {{R}_{1}} \right)$ a group of exponent $2$ , ${{R}_{2}}$ is zero or a subdirect product of 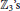${{\mathbb{Z}}_{3}}^{,}s$, and 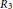${{R}_{3}}$ is zero or a subdirect product of 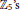${{\mathbb{Z}}_{5}}^{,}s$.