No CrossRef data available.
Article contents
An Upper Limit Property of the Euler Function
Published online by Cambridge University Press: 20 November 2018
Extract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
If ϕ(n) denotes the Euler function, for n = p a prime we have ϕ(n)/n = (1-1/p), which implies that
In this note we consider a refinement of this result. Namely, we prove that
1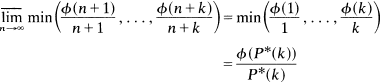
where P∗(k) is the largest integer of the form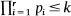 
where p1 < p2<…<pr are the first r primes in ascending order.
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1980
References
1.
Hardy, G. H., and Wright, E. M., An Introduction to the Theory of Numbers, Oxford University Press, 1968, p. 351.Google Scholar
You have
Access