Published online by Cambridge University Press: 15 November 2019
We strengthen, in various directions, the theorem of Garnett that every  $\unicode[STIX]{x1D70E}$-compact, completely regular space
$\unicode[STIX]{x1D70E}$-compact, completely regular space 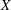 $X$ occurs as a Gleason part for some uniform algebra. In particular, we show that the uniform algebra can always be chosen so that its maximal ideal space contains no analytic discs. We show that when the space
$X$ occurs as a Gleason part for some uniform algebra. In particular, we show that the uniform algebra can always be chosen so that its maximal ideal space contains no analytic discs. We show that when the space 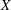 $X$ is metrizable, the uniform algebra can be chosen so that its maximal ideal space is metrizable as well. We also show that for every locally compact subspace
$X$ is metrizable, the uniform algebra can be chosen so that its maximal ideal space is metrizable as well. We also show that for every locally compact subspace 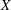 $X$ of a Euclidean space, there is a compact set
$X$ of a Euclidean space, there is a compact set 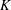 $K$ in some
$K$ in some 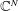 $\mathbb{C}^{N}$ so that
$\mathbb{C}^{N}$ so that 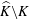 $\widehat{K}\backslash K$ contains a Gleason part homeomorphic to
$\widehat{K}\backslash K$ contains a Gleason part homeomorphic to 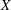 $X$, and
$X$, and 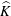 $\widehat{K}$ contains no analytic discs.
$\widehat{K}$ contains no analytic discs.
Author A.I. was partially supported by a Simons collaboration grant and by NSF Grant DMS-1856010. Author D.P. was supported by the Fonds de la Recherche Scienti que-FNRS, grant no. PDR T.0164.16