No CrossRef data available.
 $\boldsymbol {L}$-FUNCTIONS
$\boldsymbol {L}$-FUNCTIONSPublished online by Cambridge University Press: 21 March 2022
In this paper, we study the extreme values of the Rankin–Selberg L-functions associated with holomorphic cusp forms in the vertical direction. Assuming the generalised Riemann hypothesis (GRH), we prove that
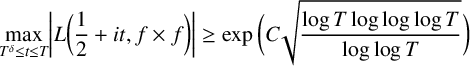 $$ \begin{align*} \underset{T^{\delta}\leq t\leq T}{\max}\bigg\lvert L\bigg(\frac12+it,f\times f\bigg)\bigg\rvert \geq\exp\bigg(C\sqrt{\frac{\log T\log\log\log T}{\log\log T}}\bigg) \end{align*} $$
$$ \begin{align*} \underset{T^{\delta}\leq t\leq T}{\max}\bigg\lvert L\bigg(\frac12+it,f\times f\bigg)\bigg\rvert \geq\exp\bigg(C\sqrt{\frac{\log T\log\log\log T}{\log\log T}}\bigg) \end{align*} $$
with
 $C\leq \mathscr {X}\sqrt {1-\delta }$
, where
$C\leq \mathscr {X}\sqrt {1-\delta }$
, where
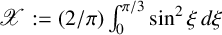 $\mathscr {X}:=({2}/{\pi })\int _{0}^{\pi /3}\sin ^2\xi \,d\xi $
and
$\mathscr {X}:=({2}/{\pi })\int _{0}^{\pi /3}\sin ^2\xi \,d\xi $
and
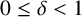 $0\leq \delta <1$
.
$0\leq \delta <1$
.
This work is supported by the Science and Technology Development Fund, Macau SAR (File No. 0066/2020/A2).