Hostname: page-component-cd9895bd7-gbm5v Total loading time: 0 Render date: 2024-12-22T10:23:23.107Z Has data issue: false hasContentIssue false
  • English
  • Français

STRUCTURE THEORY OF L(ℝ, μ) AND ITS APPLICATIONS

Published online by Cambridge University Press:  13 March 2015

NAM TRANG
NAM TRANG*
Affiliation:
DEPARTMENT OF MATHEMATICAL SCIENCES, CARNEGIE MELLON UNIVERSITY, 5000 FORBES AVE, PITTSBURGH, PA 15213, USAE-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

In this paper, we explore the structure theory of L(ℝ, μ) under the hypothesis L(ℝ, μ) ⊧ “AD + μ is a normal fine measure on 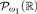” and give some applications. First we show that “ ZFC + there exist ω2 Woodin cardinals”1 has the same consistency strength as “ AD + ω1 is ℝ-supercompact”. During this process we show that if L(ℝ, μ) ⊧ AD then in fact L(ℝ, μ) ⊧ AD+. Next we prove important properties of L(ℝ, μ) including Σ1 -reflection and the uniqueness of μ in L(ℝ, μ). Then we give the computation of full HOD in L(ℝ, μ). Finally, we use Σ1 -reflection and ℙmax forcing to construct a certain ideal on 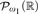(or equivalently on in this situation) that has the same consistency strength as “ZFC+ there exist ω2 Woodin cardinals.”

Keywords

Descriptive inner model theorySolovay measureHODderived modelPrikry forcingℙmax forcing
Type
Articles
Information
The Journal of Symbolic Logic , Volume 80 , Issue 1 , March 2015 , pp. 29 - 55
Copyright
Copyright © The Association for Symbolic Logic 2015 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Cummings, J., Iterated forcing and elementary embeddings, Handbook of Set Theory, pp. 775–883, 2010.Google Scholar
Ketchersid, R., Toward ADℝfrom the Continuum Hypothesis and anω 1-dense ideal, Ph. D. thesis, Berkeley, 2000.Google Scholar
Koellner, P. and Woodin, W. H., Large cardinals from determinacy, Handbook of Set Theory, pp. 1951–2119, 2010.CrossRefGoogle Scholar
Larson, Paul B., The stationary tower: Notes on a course by W. Hugh Woodin, vol. 32, University Lecture Series, American Mathematical Society, Providence, RI, 2004.Google Scholar
Larson, Paul B., Forcing over models of determinacy, Handbook of Set Theory, pp. 2121–2177, 2010.CrossRefGoogle Scholar
Sargsyan, Grigor, A tale of hybrid mice, available athttp://math.rutgers.edu/∼gs481/.Google Scholar
Schindler, Ralf and Steel, John R., The core model induction, available atmath.berkeley.edu/∼steel.Google Scholar
Solovay, R., The independence of DC from AD, Cabal Seminar 76–77, pp. 171–183. Springer, New York, 1978.Google Scholar
Steel, J. R., The derived model theorem, available atwww.math.berkeley.edu/∼steel.Google Scholar
Steel, J. R., Derived models associated to mice. Computational prospects of infinity. Part I. Tutorials, vol. 14, Lecture Notes Series, Institure of Mathematical Science, National Institure of Singapore, pp. 105–193, World Scientific, Hackensack, NJ, 2008.Google Scholar
Steel, J. R., Scales in K(ℝ). Games, scales, and Suslin cardinals. The Cabal Seminar. Vol. I, Lecture Notes in Logic, pp. 176–208, vol. 31, Association of Symbolic Logic, Chicago, IL, 2008.CrossRefGoogle Scholar
Steel, J. R., An outline of inner model theory, Handbook of Set Theory, pp. 1595–1684, 2010.Google Scholar
Steel, John R. and Woodin, Hugh W., HOD as a core model, 2012.Google Scholar
Steel, John and Zoble, Stuart, Determinacy from strong reflection. Transactions of the American Mathematical Society, vol. 366 (2014), no. 8, pp. 4443–4490.CrossRefGoogle Scholar
Steel, J. R. and Trang, N., AD+, derived models, and σ1 -reflection, available athttp://math.cmu.edu/∼namtrang. 2011.Google Scholar
Trang, N., A hierarchy of measures from ADℝ, available athttp://math.cmu.edu/∼namtrang. 2013.Google Scholar
Trang, N., Determinacy in L(ℝ, μ). Journal of Mathematical Logic, vol. 14 (2014), no. 01.Google Scholar
Wilson, T. M., Contributions to Descriptive Inner Model Theory, PhD thesis, University of California, 2012.Google Scholar
Hugh Woodin, W., The axiom of determinacy, forcing axioms, and the nonstationary ideal, de Gruyter Series in Logic and its Applications, vol. 1, Walter de Gruyter & Co., Berlin, 1999.Google Scholar
Zhu, Y., The derived model theorem II, available athttp://graduate.math.nus.edu.sg/∼g0700513/ der.pdf. 2010.Google Scholar