Hostname: page-component-586b7cd67f-tf8b9 Total loading time: 0 Render date: 2024-11-28T08:36:34.173Z Has data issue: false hasContentIssue false
  • English
  • Français

Large eddy simulation of transient upstream/downstream vortex interactions

Published online by Cambridge University Press:  09 January 2019

Kyle J. Forster Open the ORCID record for Kyle J. Forster [Opens in a new window] ,
Sammy Diasinos ,
Graham Doig  and
Tracie J. Barber
Kyle J. Forster*
Affiliation:
School of Mechanical and Maunfacturing Engineering, UNSW Sydney, NSW, 2053, Australia
Sammy Diasinos
Affiliation:
Department of Engineering, Macquarie University, North Ryde, NSW, 2109, Australia
Graham Doig
Affiliation:
School of Mechanical and Maunfacturing Engineering, UNSW Sydney, NSW, 2053, Australia Aerospace Engineering Department, California Polytechnic State University, CA 93407, USA
Tracie J. Barber
Affiliation:
School of Mechanical and Maunfacturing Engineering, UNSW Sydney, NSW, 2053, Australia
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Experimentally validated large eddy simulations were performed on two NACA0012 vanes at various lateral offsets to observe the transient effects of the near field interactions between two streamwise vortices. The vanes were separated in the streamwise direction, allowing the upstream vortex to impact on the downstream geometry. These vanes were evaluated at an angle of incidence of 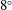$8^{\circ }$ and a Reynolds number of 70 000, with rear vane angle reversed to create a co-rotating or counter-rotating vortex pair. The downstream vortex merged with the upstream in the co-rotating condition, driven by the suppression of one of the tip vortices of the downstream vane. At close proximity to the pressure side, the vane elongated the upstream vortex, resulting in it being the weakened and merging into the downstream vortex. This produced a transient production of bifurcated vortices in the wake region. The downstream vortex of the co-rotating pair experienced faster meandering growth, with position oscillations equalising between the vortices. The position oscillation was determined to be responsible for statistical variance in the merging location, with variation in vortex separation causing the vortices at a single plane to merge and separate in a time-dependent manner. In the counter-rotating condition position oscillations were found to be larger, with higher growth, but less uniform periodicity. It was found that the circulation transfer between the vortices was linked to the magnitude of their separation, with high separation fluctuations weakening the upstream vortex and strengthening the downstream vortex. In the case of upstream vortex impingement on the downstream vane, the upstream vortex was found to bifurcate, with a four vortex system being formed by interactions with the shear layer. This eventually resulted in a single dominant vortex, which did not magnify its oscillation amplitudes as it travelled downstream due to the destruction of the interacting vortices.

JFM classification

Vortex Flows: Vortex dynamics Vortex Flows: Vortex instability Vortex Flows: Vortex interactions
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 862 , 10 March 2019 , pp. 227 - 260
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Brandt, L. K. & Nomura, K. K. 2010 Characterization of the interactions of two unequal co-rotating vortices. J. Fluid Mech. 646, 233–253.10.1017/S0022112009992849Google Scholar
Chatelain, P., Curioni, A., Bergdorf, M., Rossinelli, D., Andreoni, W. & Koumoutsakos, P. 2008 Billion vortex particle direct numerical simulations of aircraft wakes. Comput. Meth. Appl. Mech. Engng 197, 1296–1304.10.1016/j.cma.2007.11.016Google Scholar
Courant, R., Friedrichs, K. O. & Lewy, H. 1967 On the partial difference equation of mathematical physics. IBM J. 11 (March), 32–74.10.1147/rd.112.0215Google Scholar
Crow, S. C. 1970 Stability theory for a pair of trailing vortices. AIAA J. 8 (12), 2172–2179.10.2514/3.6083Google Scholar
Dacles-Mariani, J., Zilliac, G. G., Chow, J. S. & Bradshaw, P. 1995 Numerical/experimental study of a wingtip vortex in the near field. AIAA J. 33 (9), 1561–1568.10.2514/3.12826Google Scholar
Devenport, W. J., Zsoldos, J. S. & Vogel, C. M. 1997 The structure and development of a counter-rotating wing-tip vortex pair. J. Fluid Mech. 332, 71–104.10.1017/S0022112096003795Google Scholar
Dritschel, D. G. 1985 The stability and energetics of corotating uniform vortices. J. Fluid Mech. 157, 95–134.10.1017/S0022112085002324Google Scholar
Dritschel, D. G. & Waugh, D. W. 1992 Quantification of the inelastic interaction of unequal vortices in two-dimensional vortex dynamics. Phys. Fluids A 4, 1737.10.1063/1.858394Google Scholar
Fabre, D., Jacquin, L. & Loof, A. 2002 Optimal perturbations in a four-vortex aircraft wake in counter-rotating configuration. J. Fluid Mech. 451, 319–328.10.1017/S0022112001006954Google Scholar
Folz, P. J. R. & Nomura, K. K. 2017 A quantitative assessment of viscous asymmetric vortex pair interactions. J. Fluid Mech. 829, 1–30.10.1017/jfm.2017.527Google Scholar
Forster, K. J., Barber, T., Diasinos, S. & Doig, G.2015 Numerical investigation of streamwise vortex interaction. SAE Technical Paper. SAE International.Google Scholar
Forster, K. J., Barber, T., Diasinos, S. & Doig, G. 2017a The variation in co and counter-rotating upstream-downstream vortex interactions. In 47th AIAA Fluid Dynamics Conference (June), pp. 1–13.Google Scholar
Forster, K. J., Barber, T. J., Diasinos, S. & Doig, G. 2017b Interactions of a co-rotating vortex pair at multiple offsets. Phys. Fluids 29, 057102.Google Scholar
Forster, K. J., Barber, T. J., Diasinos, S. & Doig, G. 2017c Interactions of a counter-rotating vortex pair at multiple offsets. Exp. Therm. Fluid Sci. 86, 63–74.10.1016/j.expthermflusci.2017.04.007Google Scholar
Forster, K. J. & White, T. R. 2014 Numerical investigation into vortex generators on heavily cambered wings. AIAA J. 52 (5), 1059–1071.Google Scholar
Garmann, D. J. & Visbal, M. R. 2015 Interactions of a streamwise-oriented vortex with a finite wing. J. Fluid Mech. 767, 782–810.10.1017/jfm.2015.51Google Scholar
Giuni, M. & Green, R. B. 2013 Vortex formation on squared and rounded tip. Aerosp. Sci. Technol. 29 (1), 191–199.10.1016/j.ast.2013.03.004Google Scholar
Gordnier, R. E. & Visbal, M. R. 1999 Numerical simulation of the impingement of a streamwise vortex on a plate. Intl J. Comput. Fluid Dyn. 12 (1), 49–66.Google Scholar
Huang, R. F. & Lin, C. L. 1995 Vortex shedding and shear-layer instability of wing at low-Reynolds numbers. AIAA J. 33 (8), 1398–1403.Google Scholar
Hummel, D. 1995 Formation flight as an energy-saving mechanism. Israel J. Zoology 41 (3), 261–278.Google Scholar
Inasawa, A., Mori, F. & Asai, M. 2012 Detailed observations of interactions of wingtip vortices in close-formation flight. J. Aircraft 49 (1), 206–213.10.2514/1.C031480Google Scholar
Kaya, F. & Karagoz, I. 2008 Performance analysis of numerical schemes in highly swirling turbulent flows in cyclones. Curr. Sci. 94 (10), 1273–1278.Google Scholar
Klein, R. 1995 Simplified equations for the interaction of nearly parallel vortex filaments. J. Fluid Mech. 288, 201–248.10.1017/S0022112095001121Google Scholar
Legras, B. & Dritschel, D. 1993 Vortex stripping and the generation of high vorticity gradients in two-dimensional flows. Appl. Sci. Res. 51, 445–455.10.1007/BF01082574Google Scholar
Lehmkuhl, O., Rodríguez, I., Baez, A., Oliva, A. & Pérez-Segarra, C. D. 2013 On the large-Eddy simulations for the flow around aerodynamic profiles using unstructured grids. Comput. Fluids 84, 176–189.10.1016/j.compfluid.2013.06.002Google Scholar
Leweke, T., Le Dizès, S. & Williamson, C. H. K. 2016 Dynamics and instabilities of vortex pairs. Annu. Rev. Fluid Mech. 48, 507–541.10.1146/annurev-fluid-122414-034558Google Scholar
Ma, J., Wang, F. & Tang, X. 2009 Comparison of Several Subgrid-Scale Models for Large-Eddy Simulation of Turbulent Flows in Water Turbine. pp. 328–334. Springer.Google Scholar
Manolesos, M. & Voutsinas, S. G. 2015 Experimental investigation of the flow past passive vortex generators on an airfoil experiencing three-dimensional separation. J. Wind Engng Ind. Aerodyn. 142, 130–148.10.1016/j.jweia.2015.03.020Google Scholar
Meunier, P. & Leweke, T. 2005 Elliptic instability of a co-rotating vortex pair. J. Fluid Mech. 533, 125–159.10.1017/S0022112005004325Google Scholar
Nicoud, F. & Ducros, F. 1999 Subgrid-scale stress modelling based on the square of the velocity gradient tensor. Flow Turbul. Combust. 62, 183–200.10.1023/A:1009995426001Google Scholar
Overman, E. A. 1982 Evolution and merger of isolated vortex structures. Phys. Fluids 25, 1297.Google Scholar
Patankar, S. V. 1971 Numerical Heat Transfer and Fluid Flow. McGraw-Hill Book Company.Google Scholar
Pereira, L. A. A., Hirata, M. H. & Filho, N. M. 2004 Wake and aerodynamics loads in multiple bodies-application to turbomachinery blade rows. J. Wind Engng Ind. Aerodyn. 92, 477–491.10.1016/j.jweia.2004.02.001Google Scholar
Peyret, R. 1996 Handbook of Computational Fluid Mechanics. Academic Press.Google Scholar
Probst, A. & Reuß, S. 2015 Scale-Resolving Simulations of Wall-Bounded Flows with an Unstructured Compressible Flow Solver. pp. 481–491. Springer International Publishing.Google Scholar
Roberts, K. V. & Christiansen, J. P. 1972 Topics in computational fluid dynamics. Comput. Phys. Commun. 3 (I 972), 14–32.10.1016/0010-4655(72)90111-7Google Scholar
Rokhsaz, K. & Kliment, L. K. 2002 Experimental investigation of co-rotating vortex filaments in a water tunnel. In 32nd AIAA Fluid Dynamics Conference and Exhibit, vol. 40, pp. 1115–1122.Google Scholar
Safdari, A. & Kim, K. C. 2015 Aerodynamic and structural evaluation of horizontal archimedes spiral wind turbine. J. Clean Energy Technol. 3 (1), 34–38.10.7763/JOCET.2015.V3.164Google Scholar
Toloui, M., Chamorro, L. P. & Hong, J. 2015 Detection of tip-vortex signatures behind a 2.5 MW wind turbine. J. Wind Engng Ind. Aerodyn. 143, 105–112.10.1016/j.jweia.2015.05.001Google Scholar
Trieling, R. R. & Van Heijst, G. J. F. 1998 Kinematic properties of monopolar vortices in a strain flow. Fluid Dyn. Res. 23, 319–341.10.1016/S0169-5983(97)00053-1Google Scholar
Tsai, C.-Y. & Widnall, S. E. 1976 The stability of short waves on a straight vortex filament in a weak externally imposed strain field. J. Fluid Mech. 73, 721–733.10.1017/S0022112076001584Google Scholar
Uzun, A. & Hussaini, M. Y. 2010 Simulations of vortex formation around a blunt wing tip. AIAA J. 48 (6), 1221–1234.10.2514/1.J050147Google Scholar
Van Driest, E. R. 1956 On turbulent flow near a wall. J. Aero. Sci. 23 (4), 1007–1011.Google Scholar
Widnall, S. E. 1975 The structure and dynamics of vortex filaments. Annu. Rev. Fluid Mech. 7, 141–165.10.1146/annurev.fl.07.010175.001041Google Scholar
Yilmaz, I. & Davidson, L. 2015 Comparison of SGS models in Large-Eddy Simulation for transition to turbulence in Taylor–Green flow. In The 16th International Conference on Fluid Flow Technologies CMFF 2015, Budapest, Hungary.Google Scholar