Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Zampogna, Giuseppe A.
Naqvi, Sahrish B.
Magnaudet, Jacques
and
Bottaro, Alessandro
2019.
Compliant riblets: Problem formulation and effective macrostructural properties.
Journal of Fluids and Structures,
Vol. 91,
Issue. ,
p.
102708.
Ledda, P. G.
Siconolfi, L.
Viola, F.
Camarri, S.
and
Gallaire, F.
2019.
Flow dynamics of a dandelion pappus: A linear stability approach.
Physical Review Fluids,
Vol. 4,
Issue. 7,
Bottaro, Alessandro
2019.
Flow over natural or engineered surfaces: an adjoint homogenization perspective.
Journal of Fluid Mechanics,
Vol. 877,
Issue. ,
Zhu, Guangpu
Kou, Jisheng
Yao, Bowen
Wu, Yu-shu
Yao, Jun
and
Sun, Shuyu
2019.
Thermodynamically consistent modelling of two-phase flows with moving contact line and soluble surfactants.
Journal of Fluid Mechanics,
Vol. 879,
Issue. ,
p.
327.
Dewangan, Mainendra Kumar
and
Datta, Subhra
2019.
Improved asymptotic predictions for the effective slip over a corrugated topography.
Applied Mathematical Modelling,
Vol. 72,
Issue. ,
p.
247.
Picella, Francesco
Robinet, J.-Ch.
and
Cherubini, S.
2019.
Laminar–turbulent transition in channel flow with superhydrophobic surfaces modelled as a partial slip wall.
Journal of Fluid Mechanics,
Vol. 881,
Issue. ,
p.
462.
Bottaro, Alessandro
and
Naqvi, Sahrish B.
2020.
Effective boundary conditions at a rough wall: a high-order homogenization approach.
Meccanica,
Vol. 55,
Issue. 9,
p.
1781.
Picella, F.
Robinet, J.-Ch.
and
Cherubini, S.
2020.
On the influence of the modelling of superhydrophobic surfaces on laminar–turbulent transition.
Journal of Fluid Mechanics,
Vol. 901,
Issue. ,
Xiong, Xiangming
and
Tao, Jianjun
2020.
Linear stability and energy stability of plane Poiseuille flow with isotropic and anisotropic slip boundary conditions.
Physics of Fluids,
Vol. 32,
Issue. 9,
Lācis, Uǧis
Sudhakar, Y.
Pasche, Simon
and
Bagheri, Shervin
2020.
Transfer of mass and momentum at rough and porous surfaces.
Journal of Fluid Mechanics,
Vol. 884,
Issue. ,
Zhang, Chengpeng
Jiang, Zhaoliang
Wang, Jilai
Zhao, Li
Wang, Ding
and
Zhang, Cheng
2020.
Theoretical modeling and experimental characterization of boundary slip on aluminum surface with small-amplitude roughness.
Engineering Research Express,
Vol. 2,
Issue. 1,
p.
015025.
Zuo, Hong
Javadpour, Farzam
Deng, Shouchun
and
Li, Haibo
2020.
Liquid slippage on rough hydrophobic surfaces with and without entrapped bubbles.
Physics of Fluids,
Vol. 32,
Issue. 8,
Zampogna, Giuseppe A.
and
Gallaire, François
2020.
Effective stress jump across membranes.
Journal of Fluid Mechanics,
Vol. 892,
Issue. ,
Li, Zexiang
Ji, Songsong
Duan, Huiling
Lan, Shilong
Zhang, Jinbai
and
Lv, Pengyu
2020.
Coupling effect of wall slip and spanwise oscillation on drag reduction in turbulent channel flow.
Physical Review Fluids,
Vol. 5,
Issue. 12,
Sudhakar, Y.
Lācis, Ugis
Pasche, Simon
and
Bagheri, Shervin
2021.
Higher-Order Homogenized Boundary Conditions for Flows Over Rough and Porous Surfaces.
Transport in Porous Media,
Vol. 136,
Issue. 1,
p.
1.
Ledda, Pier Giuseppe
Boujo, E.
Camarri, S.
Gallaire, F.
and
Zampogna, G.A.
2021.
Homogenization-based design of microstructured membranes: wake flows past permeable shells.
Journal of Fluid Mechanics,
Vol. 927,
Issue. ,
Ciuti, M.
Zampogna, G. A.
Gallaire, F.
Camarri, S.
and
Ledda, P. G.
2021.
On the effect of a penetrating recirculation region on the bifurcations of the flow past a permeable sphere.
Physics of Fluids,
Vol. 33,
Issue. 12,
Tian, Guizhong
Fan, Dongliang
Feng, Xiaoming
and
Zhou, Honggen
2021.
Thriving artificial underwater drag-reduction materials inspired from aquatic animals: progresses and challenges.
RSC Advances,
Vol. 11,
Issue. 6,
p.
3399.
Valdés-Parada, F. J.
and
Lasseux, D.
2021.
A novel one-domain approach for modeling flow in a fluid-porous system including inertia and slip effects.
Physics of Fluids,
Vol. 33,
Issue. 2,
Shan, Baochao
Wang, Peng
Wang, Runxi
Zhang, Yonghao
and
Guo, Zhaoli
2022.
Molecular kinetic modelling of nanoscale slip flow using a continuum approach.
Journal of Fluid Mechanics,
Vol. 939,
Issue. ,
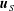 $\boldsymbol{u}_{S}$ on an equivalent (smooth) surface in the form
$\boldsymbol{u}_{S}$ on an equivalent (smooth) surface in the form 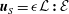 $\boldsymbol{u}_{S}=\unicode[STIX]{x1D716}{\mathcal{L}}\boldsymbol{ : }{\mathcal{E}}$, where the dimensionless parameter
$\boldsymbol{u}_{S}=\unicode[STIX]{x1D716}{\mathcal{L}}\boldsymbol{ : }{\mathcal{E}}$, where the dimensionless parameter  $\unicode[STIX]{x1D716}$ is a measure of the roughness amplitude,
$\unicode[STIX]{x1D716}$ is a measure of the roughness amplitude,  ${\mathcal{E}}$ denotes the strain-rate tensor associated with the outer flow in the vicinity of the surface and
${\mathcal{E}}$ denotes the strain-rate tensor associated with the outer flow in the vicinity of the surface and  ${\mathcal{L}}$ is a third-order slip tensor arising from the microscopic geometry characterizing the rough surface. This boundary condition represents the tensorial generalization of the classical Navier slip condition. We derive this condition, in the limit of small microscopic Reynolds numbers, using a multi-scale technique that yields a closed system of equations, the solution of which allows the slip tensor to be univocally calculated, once the roughness geometry is specified. We validate this generalized slip condition by considering the flow about a rough sphere, the surface of which is covered with a hexagonal lattice of cylindrical protrusions. Comparisons with direct numerical simulations performed in both laminar and turbulent regimes allow us to assess the validity and limitations of this condition and of the mathematical model underlying the determination of the slip tensor
${\mathcal{L}}$ is a third-order slip tensor arising from the microscopic geometry characterizing the rough surface. This boundary condition represents the tensorial generalization of the classical Navier slip condition. We derive this condition, in the limit of small microscopic Reynolds numbers, using a multi-scale technique that yields a closed system of equations, the solution of which allows the slip tensor to be univocally calculated, once the roughness geometry is specified. We validate this generalized slip condition by considering the flow about a rough sphere, the surface of which is covered with a hexagonal lattice of cylindrical protrusions. Comparisons with direct numerical simulations performed in both laminar and turbulent regimes allow us to assess the validity and limitations of this condition and of the mathematical model underlying the determination of the slip tensor 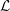 ${\mathcal{L}}$.
${\mathcal{L}}$.