Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Shen, Xiaoteng
Lin, Mingze
Zhu, Yuliang
Ha, Ho Kyung
Fettweis, Michael
Hou, Tianfeng
Toorman, Erik A.
Maa, Jerome P.-Y.
and
Zhang, Jinfeng
2021.
A quasi-Monte Carlo based flocculation model for fine-grained cohesive sediments in aquatic environments.
Water Research,
Vol. 194,
Issue. ,
p.
116953.
Yao, Yuan
and
Capecelatro, Jesse
2021.
Deagglomeration of cohesive particles by turbulence.
Journal of Fluid Mechanics,
Vol. 911,
Issue. ,
Zhao, K.
Pomes, F.
Vowinckel, B.
Hsu, T.-J.
Bai, B.
and
Meiburg, E.
2021.
Flocculation of suspended cohesive particles in homogeneous isotropic turbulence.
Journal of Fluid Mechanics,
Vol. 921,
Issue. ,
Vowinckel, Bernhard
2021.
Incorporating grain-scale processes in macroscopic sediment transport models.
Acta Mechanica,
Vol. 232,
Issue. 6,
p.
2023.
Chen, Pinzhuo
Chen, Sheng
Yang, Mengmeng
and
Li, Shuiqing
2021.
Falling clouds of particles with finite inertia in viscous flows.
Physics of Fluids,
Vol. 33,
Issue. 3,
Fu, Jianhong
Chen, Sheng
and
Zhou, Xiaochen
2022.
Effect of heterogeneity on interphase heat transfer for gas–solid flow: A particle-resolved direct numerical simulation.
Physics of Fluids,
Vol. 34,
Issue. 12,
Vowinckel, Bernhard
Zhao, Kunpeng
Ye, Leiping
J. Manning, Andrew
Hsu, Tian-Jian
Meiburg, Eckart
and
Bai, Bofeng
2022.
Sediment Transport - Recent Advances.
Zhu, Rui
He, Zhiguo
Zhao, Kunpeng
Vowinckel, Bernhard
and
Meiburg, Eckart
2022.
Grain-resolving simulations of submerged cohesive granular collapse.
Journal of Fluid Mechanics,
Vol. 942,
Issue. ,
Yu, Minglan
Yu, Xiao
and
Balachandar, S.
2022.
Particle Nonresolved DNS‐DEM Study of Flocculation Dynamics of Cohesive Sediment in Homogeneous Isotropic Turbulence.
Water Resources Research,
Vol. 58,
Issue. 6,
Chen, Sheng
Chen, Pinzhuo
and
Fu, Jianhong
2022.
Drag and lift forces acting on linear and irregular agglomerates formed by spherical particles.
Physics of Fluids,
Vol. 34,
Issue. 2,
Muñoz-Royo, Carlos
Ouillon, Raphael
El Mousadik, Souha
Alford, Matthew H.
and
Peacock, Thomas
2022.
An in situ study of abyssal turbidity-current sediment plumes generated by a deep seabed polymetallic nodule mining preprototype collector vehicle.
Science Advances,
Vol. 8,
Issue. 38,
Zhu, Zhongfan
2022.
Predicting the flocculation kinetics of fine particles in a turbulent flow using a Budyko-type model.
Environmental Science and Pollution Research,
Vol. 29,
Issue. 56,
p.
84268.
Vowinckel, Bernhard
Zhao, Kunpeng
Zhu, Rui
and
Meiburg, Eckart
2023.
Investigating cohesive sediment dynamics in open waters via grain-resolved simulations.
Flow,
Vol. 3,
Issue. ,
Zhao, K.
Vowinckel, B.
Hsu, T.-J.
Bai, B.
and
Meiburg, E.
2023.
Cohesive sediment: intermediate shear produces maximum aggregate size.
Journal of Fluid Mechanics,
Vol. 965,
Issue. ,
Zhao, Kunpeng
Zhao, Shuaiqi
Huang, Han
Zhang, Rui
Meiburg, Eckart
and
Bai, Bofeng
2024.
Flocculation rate of locally densely distributed cohesive particles in Taylor–Green vortex flow.
Physics of Fluids,
Vol. 36,
Issue. 4,
Zhu, Rui
He, Zhiguo
and
Meiburg, Eckart
2024.
Effects of initial packing density and cohesion on submerged granular collapse.
Physical Review Fluids,
Vol. 9,
Issue. 8,
Zhao, K.
Huang, H.
Zhao, S.
Pei, B.
Meiburg, E.
and
Bai, B.
2024.
Intermediate temperature of supercritical water enhances the dispersion of cohesive particles.
Chemical Engineering Science,
Vol. 286,
Issue. ,
p.
119659.
Chen, Yu
Suo, Si
Dong, Mingrui
Zhong, Haiyi
Wei, Deheng
and
Gan, Yixiang
2024.
Effects of particle density and fluid properties on mono-dispersed granular flows in a rotating drum.
Physics of Fluids,
Vol. 36,
Issue. 10,
Zhao, Shuaiqi
Zhang, Rui
Huang, Han
Zhao, Kunpeng
and
Bai, Bofeng
2024.
Chemical reaction of solid particle aggregates in quiescent fluids.
Chemical Engineering Journal,
Vol. 501,
Issue. ,
p.
157709.
Zhao, Junguang
Ding, Yun
Zhang, Tao
and
Yang, Qing
2025.
Investigating the impact of cruciform stepped spoiler bars on floc formation and flocculation kinetics parameters.
Separation and Purification Technology,
Vol. 358,
Issue. ,
p.
130371.
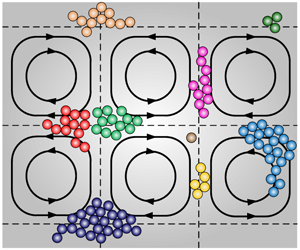
 $O(1)$. Results from this simple computational model are consistent with experimental observations, thus allowing us to propose a new analytical flocculation model that yields improved agreement with experimental data, especially during the transient stages.
$O(1)$. Results from this simple computational model are consistent with experimental observations, thus allowing us to propose a new analytical flocculation model that yields improved agreement with experimental data, especially during the transient stages.