Hostname: page-component-586b7cd67f-t7fkt Total loading time: 0 Render date: 2024-12-01T00:29:50.175Z Has data issue: false hasContentIssue false
  • English
  • Français

Large eddy simulation of flow around a reverse rotating propeller

Published online by Cambridge University Press:  18 July 2013

Hyunchul Jang  and
Krishnan Mahesh
Hyunchul Jang
Affiliation:
Aerospace Engineering & Mechanics, University of Minnesota, Minneapolis, MN 55455, USA
Krishnan Mahesh*
Affiliation:
Aerospace Engineering & Mechanics, University of Minnesota, Minneapolis, MN 55455, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

This paper studies the flow around a propeller rotating in the reverse direction in a uniform free stream. Large eddy simulation is used to study this massively separated flow at a Reynolds number of 480 000 and advance ratios $J= - 0. 5$, 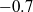$- 0. 7$ and 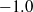$- 1. 0$. Simulations are performed on two grids; statistics of the loads and velocity field around the propeller show encouraging agreement between the two grids and with experiment. The impact of advance ratio is discussed, and a physical picture of the unsteady flow and its influence on the propeller loads is proposed. An unsteady vortex ring is formed in the vicinity of the propeller disk due to the interaction between the free stream and the reverse flow produced by the reverse rotation. The flow is separated in the blade passages; the most prominent is the separation along the sharp edge of the blade on the downstream side of the blade. This separation results in high-amplitude, transient propeller loads. Conditional averaging is used to describe the statistically relevant events that determine low- and high-amplitude thrust and side-forces. The vortex ring is closer and the reverse flow induced by propeller rotation is lower when the loads are high. The propeller loads scale with 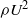$\rho {U}^{2} $ for 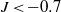$J\lt - 0. 7$ and with 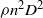$\rho {n}^{2} {D}^{2} $ for 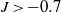$J\gt - 0. 7$.

JFM classification

Turbulent Flows: Turbulence simulation Turbulent Flows: Turbulent Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 729 , 25 August 2013 , pp. 151 - 179
Copyright
©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Amiet, R. K. 1988 Thickness noise of a propeller and its relation to blade sweep. J. Fluid Mech. 192, 535–560.Google Scholar
Antonia, R. A. 1981 Conditional sampling in turbulence measurement. Annu. Rev. Fluid Mech. 13, 131–156.Google Scholar
Beddhu, M., Taylor, L. K. & Whitfield, D. L. 1996 Strong conservative form of the incompressible Navier–Stokes equations in a rotating frame with a solution procedure. J. Comput. Phys. 128, 427–437.Google Scholar
Berchiche, N. 2008 Numerical predictions of crashback propeller flow and loadings. In Proceedings of the 8th International Conference on Hydrodynamics. China Ocean Press.Google Scholar
Bernero, S. 2000 A turbulent jet in counterflow. PhD thesis, Technische Universität Berlin.Google Scholar
Bridges, D. H., Donnelly, M. J. & Park, T. J. 2005 Experimental investigation of the submarine crashback maneuver. In 43rd AIAA Aerospace Sciences Meeting and Exhibit, Reno, Nevada. American Institute of Aeronautics & Astronautics.Google Scholar
Bridges, D. H., Donnelly, M. J. & Park, T. J. 2008 Experimental investigation of the submarine crashback maneuver. J. Fluids Engng 130.CrossRefGoogle Scholar
Chang, P., Ebert, M., Young, Y. L., Liu, Z., Mahesh, K., Jang, H. & Shearer, M. 2008 Propeller forces and structural responses to crashback. In Proceedings of the 27th Symposium on Naval Hydrodynamics, Seoul, Korea. Curran Associates Inc.Google Scholar
Chapman, C. J. 1988 Shocks and singularities in the pressure field of a supersonically rotating propeller. J. Fluid Mech. 192, 1–16.CrossRefGoogle Scholar
Chen, B. & Stern, F. 1999 Computational fluid dynamics of four quadrant marine propeller flow. J. Ship Res. 43 (4), 218–228.CrossRefGoogle Scholar
Cooper, A. J. & Peake, N. 2005 Upstream-radiated rotor–stator interaction noise in mean swirling flow. J. Fluid Mech. 523, 219–250.CrossRefGoogle Scholar
Davoudzadeh, F., Taylor, L. K., Zierke, W. C., Dreyer, J. J., McDonald, H. & Whitfield, D. L. 1997 Coupled Navier–Stokes and equations of motion simulation of submarine maneuvers, including crashback. In Proceedings of the 1997 ASME Fluids Engineering Division Summer Meeting, New York. American Society of Mechanical Engineer.Google Scholar
Donnelly, M., Jessup, S. & Etebari, A. 2008 Measurement of steady and unsteady duct loads for propeller 4381 at crashback conditions in the 36 water tunnel. Tech. Rep. NSWCCD-50-TR-2008. Naval Surface Warfare Center.Google Scholar
Drees, J. M. & Hendal, W. P. 1951 Airflow patterns in the neighbourhood of helicopter rotors: a description of some smoke tests carried out in a wind-tunnel at Amsterdam. Aircraft Engineering and Aerospace Technology 23 (4), 107–111.CrossRefGoogle Scholar
Duttweiler, M. E. & Brennen, C. E. 2002 Surge instability on a cavitating propeller. J. Fluid Mech. 458, 133–152.Google Scholar
Ebert, M., Chang, P. & Mulvihill, L. 2007 NSWCCD FY07 crashback computational effort. In ONR Propulsor S & T Program Review, October 2007.Google Scholar
Felli, M., Di Felice, F., Guj, G. & Camussi, R. 2006 Analysis of the propeller wake evolution by pressure and velocity phase measurements. Exp. Fluids 41, 441–451.Google Scholar
Furuya, O. 1985 A performance-prediction theory for partially submerged ventilated propellers. J. Fluid Mech. 151, 311–335.Google Scholar
Germano, M., Piomelli, U., Moin, P. & Cabot, W. H. 1991 A dynamic subgrid-scale eddy viscosity model. Phys. Fluids A 3 (7), 1760–1765.Google Scholar
Green, R. B., Gillies, E. A. & Brown, R. E. 2005 The flow field around a rotor in axial descent. J. Fluid Mech. 534, 237–261.Google Scholar
Hampton, G. A. 1995 Open water force and moment characteristics on three propellers in a crashback condition. Tech. Rep. CRDKNSWC/HD-1126-NV. David Taylor Naval Ship Research and Development Center.Google Scholar
Hecker, R. & Remmers, K. 1971 Four quadrant open-water performance of propellers 3710, 4024, 4086, 4381, 4382, 4383, 4384 and 4426. Tech. Rep. PNSRADC 417-H01. David Taylor Naval Ship Research and Development Center.Google Scholar
Jessup, S., Chesnakas, C., Fry, D., Donnelly, M., Black, S. & Park, J. 2004 Propeller performance at extreme off design conditions. In Proceedings of the 25th Symposium on Naval Hydrodynamics, St. John’s, Canada. National Academies Press.Google Scholar
Jessup, S., Fry, D. & Donnelly, M. 2006 Unsteady propeller performance in crashback conditions with and without duct. In Proceedings of the 26th Symposium on Naval Hydrodynamics, Rome, Italy. National Academies Press.Google Scholar
Jiang, C. W., Dong, R. R., Lui, H. L. & Chang, M. S. 1997 24-inch water tunnel flow field measurements during propeller crashback. In Proceedings of the 21st Symposium on Naval Hydrodynamics, Trondheim, Norway. National Academies Press.Google Scholar
Kinnas, S. A. & Fine, N. E. 1993 A numerical nonlinear analysis of the flow around two- and three-dimensional partially cavitating hydrofoils. J. Fluid Mech. 254, 151–181.Google Scholar
Leishman, J. G. 2006 Principles of Helicopter Aerodynamics, 2nd edn. Cambridge University Press.Google Scholar
Lilly, D. K. 1992 A proposed modification of the Germano subgrid-scale closure model. Phys. Fluids A 4 (3), 633–635.Google Scholar
Mahesh, K., Constantinescu, G. & Moin, P. 2004 A numerical method for large-eddy simulation in complex geometries. J. Comput. Phys. 197 (1), 215–240.Google Scholar
Majety, K. S. 2003 Solutions to the Navier–Stokes equations in non-inertial reference frame. Master’s thesis, Mississippi State University.Google Scholar
Niazi, S. 2000 Numerical simulation of rotating stall and surge alleviation in axial compressors. PhD thesis, Georgia Institute of Technology.Google Scholar
Pampreen, R. C. 1993 Compressor Surge and Stall. Concepts ETI, Inc.Google Scholar
Parry, A. B. 1995 The effect of blade sweep on the reduction and enhancement of supersonic propeller noise. J. Fluid Mech. 293, 181–206.Google Scholar
Shariff, K. & Leonard, A. 1992 Vortex rings. Annu. Rev. Fluid Mech. 24, 235–279.CrossRefGoogle Scholar
Staubli, T., Gyarmathy, G. & Inderbitzen, A. Visualization of rotating stall in a full size water model of a single-stage centrifugal compressor. La Houille Blanche 3/4, 40–45.Google Scholar
Tam, C. K. W. & Salikuddin, M. 1986 Weakly nonlinear acoustic and shock-wave theory of the noise of advanced high-speed turbopropellers. J. Fluid Mech. 164, 127–154.Google Scholar
Verma, A., Jang, H. & Mahesh, K. 2012 Large eddy simulation of flow around a reverse rotating propeller. J. Fluid Mech. 704, 61–88.Google Scholar
Vyšohlid, M. & Mahesh, K. 2006 Large eddy simulation of crashback in marine propellers. In Proceedings of the 26th Symposium on Naval Hydrodynamics, Rome, Italy. National Academies Press.CrossRefGoogle Scholar
Vyšohlid, M. & Mahesh, K. 2007 Understanding crashback in marine propellers using an unsteady actuator disk model. In 45th AIAA Aerospace Sciences Meeting and Exhibit, Reno, Nevada. American Institute of Aeronautics & Astronautics.Google Scholar
Yoda, M. & Fiedler, H. E. 1996 The round jet in a uniform counterflow: flow visualization and mean concentration measurements. Exp. Fluids 21, 427–436.Google Scholar