VARIATIONS ON A THEOREM OF DAVENPORT CONCERNING ABUNDANT NUMBERS

Abstract

Let 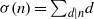$\sigma (n)= {\mathop{\sum }\nolimits}_{d\mid n} d$ be the usual sum-of-divisors function. In 1933, Davenport showed that 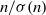$n/ \sigma (n)$ possesses a continuous distribution function. In other words, the limit 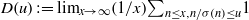$D(u): = \lim _{x\rightarrow \infty }(1/ x){\mathop{\sum }\nolimits}_{n\leq x, n/ \sigma (n)\leq u} 1$ exists for all $u\in [0, 1] $ and varies continuously with $u$. We study the behaviour of the sums 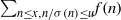${\mathop{\sum }\nolimits}_{n\leq x, n/ \sigma (n)\leq u} f(n)$ for certain complex-valued multiplicative functions $f$. Our results cover many of the more frequently encountered functions, including 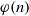$\varphi (n)$, 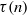$\tau (n)$ and 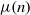$\mu (n)$. They also apply to the representation function for sums of two squares, yielding the following analogue of Davenport’s result: for all $u\in [0, 1] $, the limit

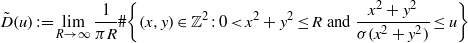$$\begin{eqnarray*}\tilde {D} (u): = \lim _{R\rightarrow \infty }\frac{1}{\pi R} \# \biggl\{ (x, y)\in { \mathbb{Z} }^{2} : 0\lt {x}^{2} + {y}^{2} \leq R\text{ and } \frac{{x}^{2} + {y}^{2} }{\sigma ({x}^{2} + {y}^{2} )} \leq u\biggr\}\end{eqnarray*}$$

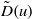exists, and $\tilde {D} (u)$ is both continuous and strictly increasing on $[0, 1] $.